Spherical waves

In an electron microscope, we have a source of electrons. Electrons fly out in all directions. We can express this using our thread/corkscrew wave analogy by attaching many threads to a point source. We assume that all the threads start off being lined up with one another, so they each start with identical phase (i.e. the white twines are lined up with adjacent white twines). Such a source is called a 'coherent emitter'. In practice, electron sources are not very coherent, but they are effectively coherent in many experimental situations (e.g. a field-emission gun in a STEM)

Our threads radiate outwards, just like the beams we drew in the alignment guide.

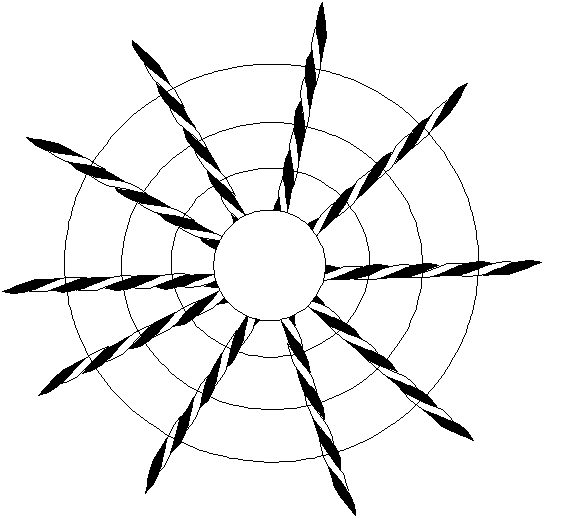
We can draw circles at distances away from the source where the threads all have their twines synchronised.

Imagine throwing a stone into a pond and watching the waves propagate outwards in circles. This is very similar to the picture above, but with one important difference. The height of a water wave is characterised by a single number: remember the value of the electron wave is a complex number.

Thinking of the water wave, we can also guess that the amplitude (or modulus) of the wave must decrease as we get further from the source. That's true, and we will have to worry about this occasionally in discussing electron waves. However, because of the sort of scales involves in real electron experiments, this amplitude term (the absolute size or radius of the electron wave/corkscrew) very rarely matters very much. What is much more important is the value of the phase of the wave, because this can make the difference between it adding positively or negatively to other waves.

To find the phase of an electron wave travelling in free space, all we have to do is tie a thread between its point of emission (or the last point where it was scattered) and then stretch the thread to the point where we want to measure its phase.

All the waves we will be concerned with are three-dimensional. To make a three-dimensional source, we need threads radiating out in all directions. Connecting points of constant phase makes a spherical surface, and many such surfaces are imbedded in one another, like the layers of an onion. Such a wave is called a spherical wave. Remember, each point in space has a complex number associated with it, which is determined by the length of the phase thread from the source to the particular point in space, which in turn determines in which direction the value of the wave is pointing in the complex plane.

The threads coming out of the source shown above will never meet up. However, in an electron microscope we have lenses and atoms that scatter the threads (rays or beams), and so eventually some threads meet up again, say at the phosphor screen. Because in general the threads (rays or beams) have taken different paths through the microscope and the specimen, they will arrive with different phases and amplitudes. At the point of measurement, we must add together all the relevant threads using the law of vector addition for their respective complex values (which, if their phases are different, will be pointing in different directions, and so some of them may cancel each other out).

Two and three-dimensional plane waves

A long way from a coherent source of electrons, like the one above, the curve of the wave fronts (the surfaces of constant phase) become straight lines in the case of two-dimensional waves, or flat surfaces (plane surfaces) in the case of three-dimensional waves. The term plane wave is the starting point of most electron microscopy. Textbooks will say "consider a specimen illuminated by a plane wave". In a plane wave, our phase threads are all parallel to one another, and the surfaces of constant phase are parallel sheets, like this:

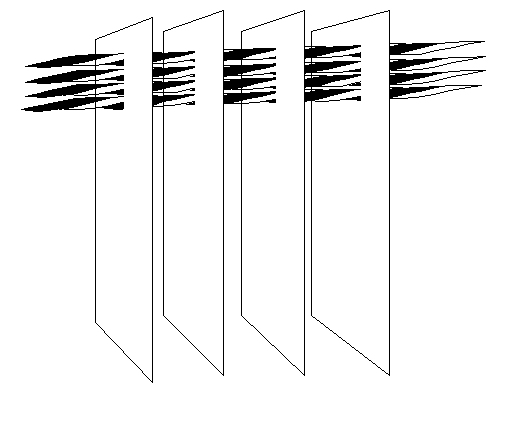
Copyright J M Rodenburg